High Resolution and High Sensitivity Imaging of Electrostatic Force
Unique Enhanced EFM Capabilities Provided Only by the Park AFM
For Electrostatic Force Microscopy (EFM), the sample surface properties investigated are electrical in nature and the interaction force used will be the electrostatic force between a biased tip and sample. However, in addition to the electrostatic force, the van der Waals forces between the tip and the sample surface are always present. The magnitude of these van der Waals forces change according to the tip-sample distance and are therefore used to measure the surface topography.
Hence the obtained signal contains both information for both surface topography and surface electrical properties generated by the van der Waals and electrostatic forces respectively. The key to successful EFM imaging lies in the separation of the EFM signal, the information regarding surface electrical properties, from the entire signal. EFM modes can be classified according to the method used to separate the EFM signal.
Enhanced EFM
Three extra EFM modes are supported by the Enhanced EFM (optional for XE Series) for Park AFM. They are Dynamic Contact Electrostatic Force Microscopy (DC-EFM), Piezoelectric Force Microscopy (PFM), and Kelvin Probe Force Microscopy (KPFM). DC-EFM, patented by Park Systems under US Patent 6,185,991, and PFM are largely identical techniques. KPFM is also known as Surface Potential Microscopy.
The schematic diagram of Enhanced EFM for Park AFM is shown in Figure 1. An external lock-in amplifier is connected to the Park AFM for two purposes. One is to apply an AC bias of frequency (ω) to the tip in addition to the DC bias being applied by the Park AFM controller. The other purpose is to separate the frequency (ω) component from the output signal. This unique capability offered only through the Enhanced EFM is what distinguishes it from the standard EFM hardware for Park AFM.
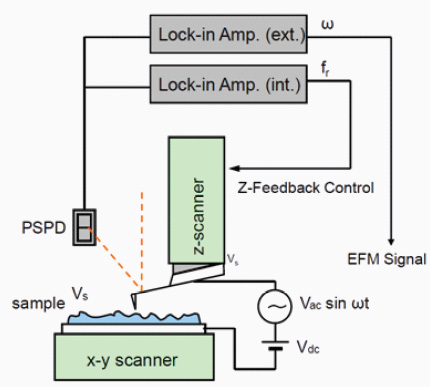Figure 1. Schematic diagram of the enhanced EFM of the Park AFM.
In the enhanced EFM, the voltage between the tip and the sample can be expressed by the following equation (1):
VDC is the DC offset potential, VS is the surface potential on the sample, and VAC and ω are the amplitude and frequency of the applied AC voltage signal, respectively.
The electrostatic force applied to the cantilever can be expressed by Equation (2), which uses the parallel plate capacitor model to describe the electrostatic interaction between the cantilever and the sample. Here, F is an electrostatic force applied to the tip, q is the charge, E is the electric field, V is the electric potential difference, C is the capacitance, and d is the tip-sample distance. Note that since both AC and DC bias are applied between the tip and the sample, three terms arise in the expression for the force between the tip and the sample. These terms can be referred to as the DC term (a), the ω term (b), and the 2ω term (c). The total cantilever deflection signal, which represents the force between the tip and sample, can be analyzed in terms of its separate parts: DC part, AC part with a frequency of ω, and AC part with a frequency of 2ω.
The DC cantilever deflection signal can be read directly from the signal channels accessible using the XEP Data Acquisition software. The AC parts of the cantilever deflection signal can be read by sending the signal to a lock-in amplifier, which can read either the part of the signal with a frequency of ω or the part of the signal with a frequency of 2ω. Together, the three signals can be used to gain information about the electrical properties of the sample. For example, the capacitance appears in the equation as the ratio of capacitance to tip-to-sample spacing, C/d. If the tip-to-sample distance is kept constant by the Z-feedback loop, then C/d is proportional to the capacitance. The ω signal, which is the coefficient of the term labeled (b) in equation (2) above, contains contributions from both C/d and the surface potential, Vs. Assuming VDC and VAC are known, you still cannot separate the contributions of the capacitance and the surface potential to the measured ω signal. However, the 2ω signal, which is the coefficient of the term labeled (c) above, only includes a contribution from the capacitance. Thus, the 2ω signal can be used to normalize the ω signal, isolating the contribution of the surface potential.
Images can be generated from any of the above-mentioned signals. Analysis of an image involves understanding each of the contributions to the signal used to generate the image.
(a) (b)
Figure 2. (a) Topography and (b) EFM phase image of the PZT film by Enhanced EFM(ext).
Why Park AFM Enhanced EFM?
Conventional EFM is conducted using unnecessary and inefficient double-pass scans, prohibitively limiting the spatial resolution of a surface potential map. Enhanced EFM for Park AFM is designed to provide efficient one-pass scans to measure both topography and surface potential simultaneously without losing spatial resolution.
• Surface charge distribution and potential imaging
• Failure analysis in micro electronics circuitry
• Mechanical hardness measurement (DC-EFM)
• Charge densitometry for ferroelectric domain
• Voltage drop on microresistors
• Work function of a semiconductor
DC-EFM
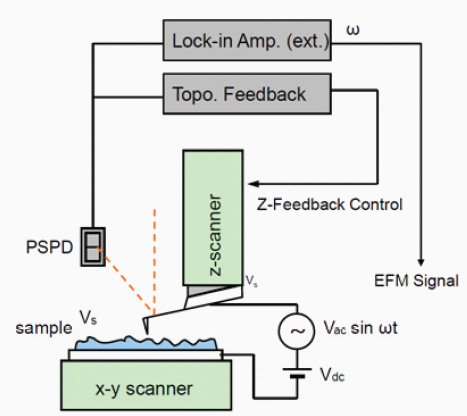
Figure 3. Schematic diagram of the Dynamic Contact EFM (DC-EFM) of the Park AFM. The unique EFM capabilities are patented and provided only by Park Systems.
DC-EFM is a mode enabled through the use of the Enhanced EFM for Park AFM that operates in Contact mode, providing much improved spatial resolution and more sensitive detection (see Figure 3). Figure 4 compares topography and surface charge images of a TGS single crystal acquired by DC-EFM (upper row) and conventional EFM (lower row). The EFM image taken with conventional EFM shows strong coupling of the topography signal to the image while the image taken with DC-EFM shows complete separation of the topography. The key advantages of DC-EFM are as follows:
• No need of special sample treatment
• High spatial resolution and noninvasive probing.
• Simultaneous topography and domain imaging (Figure 2)
• Real-time imaging of domain dynamics
• Nanoscale control and visualization of domains (Figure 5)
• Detailed local information rather than integral effect
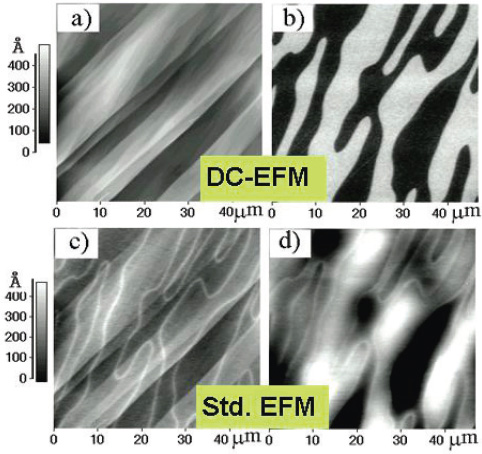
Figure 4. (a) Topography and (b) surface charge image of TGS single crystal by DC-EFM and (c) topography and (d) surface charge image by conventional EFM.
Figure 5. (a) Domain switching behavior in ferroelectric materials. Creation of small domains of TGS by (b) positive applied voltage of 10 V, and (c) negative applied voltage of 10 V.